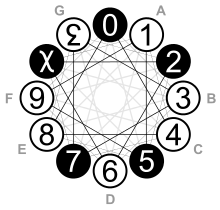
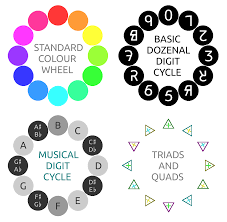
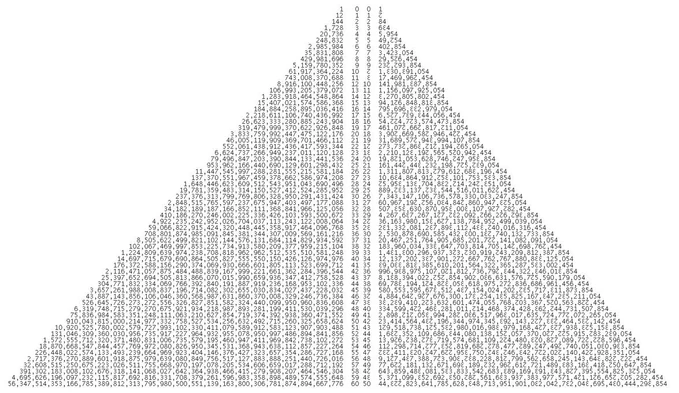
10 (twelve, dozen, onety) is the base of the numeral system of this wiki.
An interesting thing is 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + X + E + 10 + ... = −1/10 = −0.1, i.e. (this number is , which equals the negative value of the reciprocal of 10, or the additive inverse of the multiplicative inverse of 10) i.e.
The negative value of the reciprocal of 10 (i.e. −1/10) is the value counter-intuitively ascribed to the series 1+2+3+4+...
Both 10±1 are primes, both 10±2 are even semiprimes (both (10±2)/2 are primes), both 10±3 are odd semiprimes (both (10±3)/3 are primes), both 10±4 are powers of 2 (note that 4, 10−4, 10+4 are consecutive powers of 2), both 10±5 (also 10±7 and 10±E) are primes (or units), and both 10±6 are regular numbers (i.e. 3-smooth numbers) (i.e. numbers n such that 1/n terminates in dozenal) (also twice a power of 3).
Both base 10±4 are used in computing as a more compact representation of binary (1 base-8 digit per 3 bits, and 1 base-14 digit per 4 bits), these two bases are also common bases for computer.
1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + X + E + 10 + ... = −0.1 (which equals the negative value of the reciprocal of 10)
10 + 20 + 30 + 40 + 50 + 60 + 70 + 80 + 90 + X0 + E0 + 10 + ... = −1 (the sum of all positive multiples of 10 is −1)
10 is a composite number, the smallest positive integer with exactly six divisors, its divisors being 1, 2, 3, 4, 6 and 10. 10 is also a highly composite number, the next one being 20 (=2×10), and all highly composite numbers ≥10 are divisible by 10.
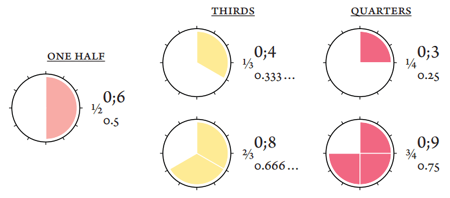
10 is the smallest base such that all of the five simplest fractions (1/2, 1/3, 2/3, 1/4, and 3/4) all only have one digit (0.6, 0.4, 0.8, 0.3, and 0.9).
10 is the smallest n such that n-th powers are squares, cubes, and biquadrates.
10 is the smallest n such that the median, the terciles, and the quartiles (i.e. all quantiles up to quartiles, quartiles are used for box plot, interquartile range (IQR), midhinge (the middle of the midsummary (trimmed midrange)), and trimean) are all n-quantiles. (the 10-quantiles are called doziles, and the median is the 6th dozile, the two terciles are the 4th and the 8th doziles, and the three quartiles are the 3rd, 6th, and 9th doziles, and 10 is the smallest such number)
10 is the smallest abundant number.
10 is the average of a twin prime pair (E and 11), i.e. both 10−1 and 10+1 are primes.
10 is the least common multiple of the n such that the complete graph Kn is planar graph: {1, 2, 3, 4}.
10 is the least common multiple of the n such that anxn+an-1xn-1+...+a1x+a0=0 have algebraic solution: {1, 2, 3, 4}.
10 is the least common multiple of the n such that the alternating group An is solvable group: {1, 2, 3, 4}.
10 is the smallest number n such that ad(n)xd(n)+ad(n)-1xd(n)-1+...+a1x+a0=0 (where d(n) is the number of positive divisors of n) does not have algebra solution.
10 is the least common multiple of the n such that regular n-gon (regular polygon with n sides) can tile the plane: {3, 4, 6}. (note that 346 is the smallest nice Friedman number, i.e. 346 = 34×6, also, 346 has terminate reciprocal, i.e. 1/346 = 0.00368, this is because 346 is regular to 10)
10 is the least common multiple of the all-harshad numbers: {1, 2, 4, 6}. (10 itself is a harshad number in all bases except base 8 (octal))
The numbers n such that all primes not dividing n are = ±1 mod n (i.e. either of the form nk+1 or of the form nk−1) are exactly the proper divisors of 10: {1, 2, 3, 4, 6}, and the least common multiple of these numbers are exactly 10.
The numbers n such that all numbers coprime to n are = ±1 mod n (i.e. either of the form nk+1 or of the form nk−1) are exactly the proper divisors of 10: {1, 2, 3, 4, 6}, and the least common multiple of these numbers are exactly 10.
The numbers n such that cos(1/n turns) is rational (in fact, 2cos(1/n turns) is integer) are exactly the proper divisors of 10: {1, 2, 3, 4, 6}, and the least common multiple of these numbers are exactly 10.
10 is the first number n such that both n and n! can be written as product of >1 factorials of primes: 10 = 2!*3! and 10! = 2!*3!*E!
10 is the only number n which is the average of 2^a and 2^b for some (a,b) pair such that a*b=n.
10 is the only number which can be written as x^y+x^(y+1) with x≥2, y≥1 in 2 different ways.
10 is the ratio of 20 and 2, where 20 is the largest n such that lambda(n)=2, and 2 is the largest n such that lambda(n)=1, where lambda is the Carmichael lambda function.
10 is the least common multiple of 1 to 4.
10 is the greatest common divisor of the order of unsolvable groups ≤ 14000.
10 is the greatest common divisor of the composite order of simple groups ≤ 14000.
10 is the product of the n such that there are no Greco-Latin squares of order n: {2, 6}.
The star numbers are exactly the centered n-gonal numbers for n=10.
10 is the value of , where is the Levy’s constant. 10 is the smallest nonnegative even number n such that the numerator of is not 1.
10 is the smallest nonnegative even number n such that the numerator of Bn has at least one prime factor ≥ n+3, where Bn is the Bernoulli number.
It is known that is irrational for n=3, and for at least one other odd n≤10.
A number n has terminate reciprocal if and only if n is regular to 10, i.e. all prime divisors of n are prime divisors of 10, besides, a number n has reciprocal with only 1 digit after the dozenal point if and only if n is divisor of 10.
10 is the only number besides 0 and 1 whose square is Fibonacci number. (interestingly, its square is the 10th Fibonacci number)
The n-th Fibonacci number F(n) has terminate reciprocal if and only if n is divisor of 10.
10 is the smallest abundant number, since it is the smallest integer for which the sum of its proper divisors (1 + 2 + 3 + 4 + 6 = 14) is greater than itself.
The centered 10-gonal (dozagonal) numbers are exactly the star numbers, since triangular numbers×n+1 are the centered n-gonal numbers, thus if we write a triangular number and add a digit “1” after it, then we get a star number.
10 is the smallest positive integer which is not power of squarefree number (i.e. not in OEIS A072774).
10 is the smallest positive integer which is neither squarefree nor prime power.
10 is the smallest positive integer which is neither squarefree nor perfect power.
10 is the smallest positive integer which is neither squarefree nor powerful.
10 is the smallest number not of the form p^e*(p - 1) for any prime p and exponent e.
10 is the smallest number n>1 such that the n-th cyclotomic polynomial has no root mod p for all primes p <= n.
10 is the smallest number k such that k/(largest power of squarefree kernel of k) is larger than 1, i.e. 10/6 = 2 > 1.
10 is the smallest number n such that (n * Product_{primes p|n} p) (OEIS A064549) is not perfect power (note that (n * Product_{primes p|n} p) is powerful for all n), and for n=10, (n * Product_{primes p|n} p) is exactly the smallest Achilles number (powerful but not perfect power) 60
The highly composite numbers contains the first 5 multiples of 10 (10, 20, 30, 40, and 50), and no other such numbers between them.
10 is the only number n such that for all prime factors p of n, p^(largest r such that p^r divides n) + p^(smallest r such that p^r does not divide n) = n. (i.e. for p=2, 2^2 + 2^3 = 10, and for p=3, 3^1 + 3^2 = 10)
If all centered m-gonal numbers are n-gonal numbers (m≥3, n≥3), then m+n = 10. (the only such (m,n) pairs are (8,4) and (9,3), note that for both these two pairs, n divides m) (note that there are no (m,n) pairs (m≥3, n≥3) such that all m-gonal numbers are centered n-gonal numbers)
10 is the only number which is both (equal to mn) and (average of 2m and 2n) for some integer pairs (m, n) such that m and n are consecutive integers (i.e. n = m+1). ((m, n) = (3, 4))
The set of 10-ominoes (dozominoes) is the lowest polyomino set in which all eight possible symmetries (four axes of reflection symmetry, aligned with the gridlines and the diagonals, and rotational symmetry of order 4; two axes of reflection symmetry, both aligned with the gridlines; two axes of reflection symmetry, both aligned with the diagonals; an axis of reflection symmetry aligned with the gridlines; an axis of reflection symmetry aligned with the diagonals; rotational symmetry of order 4; rotational symmetry of order 2; no symmetry) are realized for polyominoes without holes. (if we allow polyominoes with holes, it is the set of 8-ominoes (octominoes)) besides, the set of 10-ominoes (dozominoes) is also the lowest polyomino set in which all eight possible symmetries are realized for polyominoes which can tile the plane, besides, the set of 10-ominoes (dozominoes) is also the lowest polyomino set in which all eight possible symmetries are realized for polyominoes with holes, besides, if we allow polyominoes with holes, the number of 10-ominoes (dozominoes) with all eight possible symmetries get records for the number of n-ominoes with the same symmetry except “an axis of reflection symmetry aligned with the diagonals”. (there are totally 30980 10-ominoes (dozominoes) (including 2X135 without holes and 2847 with holes), 3 with “four axes of reflection symmetry, aligned with the gridlines and the diagonals, and rotational symmetry of order 4”, 13 with “two axes of reflection symmetry, both aligned with the gridlines”, 3 with “two axes of reflection symmetry, both aligned with the diagonals”, 245 with “an axis of reflection symmetry aligned with the gridlines”, 67 with “an axis of reflection symmetry aligned with the diagonals”, 3 with “rotational symmetry of order 4”, 1E2 with “rotational symmetry of order 2”, 3047X with “no symmetry”, all get records except “an axis of reflection symmetry aligned with the diagonals”, the former records of these eight types of symmetry are 2 (by nonomino (9-omino)), X (by elpomino (E-omino)), 2 (by elpomino (E-omino)), 103 (by elpomino (E-omino)), 77 (by elpomino (E-omino)), 1 (by octomino (8-omino)), 61 (by both dekromino (X-omino) and elpomino (E-omino)), 983X (by elpomino (E-omino)), respectively, there are 77 elpominoes (E-ominoes) with this type of symmetry, note that the difference of 77 and 67 is exactly 10)
"four axes of reflection symmetry, aligned with the gridlines and the diagonals, and rotational symmetry of order 4" without hole:
oo oooo oooo oo
"two axes of reflection symmetry, both aligned with the gridlines" without hole:
oooo oooo oooo
"two axes of reflection symmetry, both aligned with the diagonals" without hole:
o o oooo oooo o o
"an axis of reflection symmetry aligned with the gridlines" without hole:
ooooooooooo
o
"an axis of reflection symmetry aligned with the diagonals" without hole:
oooooo oo o o o o
"rotational symmetry of order 4" without hole:
o o oooo oooo o o
"rotational symmetry of order 2" without hole:
oooooo
oooooo
"no symmetry" without hole:
ooooooooooo o
"four axes of reflection symmetry, aligned with the gridlines and the diagonals, and rotational symmetry of order 4" with hole:
o ooo oo oo ooo o
"two axes of reflection symmetry, both aligned with the gridlines" with hole:
ooooo o o ooooo
"two axes of reflection symmetry, both aligned with the diagonals" with hole":
o oooo o o oooo o
"an axis of reflection symmetry aligned with the gridlines" with hole:
ooo o ooooo ooo
"an axis of reflection symmetry aligned with the diagonals" with hole:
ooo o o ooooo o o
"rotational symmetry of order 4" with hole:
o oooo o o oooo o
"rotational symmetry of order 2" with hole:
oo ooo o o ooo oo
"no symmetry" with hole:
ooooooo o o ooo
10 is the number of 5-ominoes (pentominoes), and the set of 5-ominoes (pentominoes) is the only set besides trivial 0-ominoes, 1-ominoes, and 2-ominoes (all of which have only one such polyominoes) which can tile a rectangle.
10 is the largest number n such that if 0≤k≤n−1 and gcd(k, n) = 1 (i.e. k and n are coprime), then n+k is prime.
Both of 10−1 and 10+1 are primes, in fact, all of 10±1, (10/2)±1, and (10/3)±1 are primes, the next such number is 220990, besides, all such numbers must be divisible by 10.
10 is the sum of the numbers in the first Pythagorean triple: {3, 4, 5}.
All products of three numbers in Pythagorean triple are divisible by 10.
10−1 is the largest number which cannot be written as sum of two composite numbers, 10+1 is the largest number which cannot be written as sum of a positive squarefree number and a positive square, the average of them is 10.
10−2 is the smallest noncototient, 10+2 is the smallest nontotient, the average of them is 10.
10−1 is the smallest Mirimanoff prime (generalized Wieferich prime to base 3), 10+1 is the smallest Pell-Wieferich prime (generalized Wall-Sun-Sun prime to Pell numbers), the average of them is 10.
10−2 is the length of the longest cycle in “sum of square of digits”, 10+2 is the length of the longest cycle in “sum of cube of digits”, the average of them is 10. (note that the smallest number in these two cycles are both 8)
10 is the average of the smallest nontotient (12) and the smallest noncototient (X).
10 is the first sublime number, a number that has a perfect number (6) of divisors, and the sum of its divisors is also a perfect number (24), there are only two known such numbers (including 10 itself).
10 is the product of the first three factorials (not including 0! = 1).
10 is the smallest base b such that the number of trailing zeros in n! (the factorial of n) does not depend on one of the primes or prime powers dividing b.
The square of any prime >3 is congruent to 1 mod 10 (in fact, congruent to 1 mod 20).
The sum of any pair of twin primes (other than 3 and 5) is divisible by 10. (i.e. end with 0)
The product of any pair of twin primes (other than 3 and 5) is congruent to E mod 10. (i.e. end with E)
10 is the largest number which is a divisor of the sum of all pairs of twin primes other than (3, 5).
10 is the largest number which is a divisor of the sum of all but finitely many pairs of twin primes (assuming that there are infinitely many pairs of twin primes).
10 is the smallest number n such that there exists Pythagorean triple {x, y, z} such that x+y+z = n.
10 is the largest number which is a divisor of xy for all Pythagorean triples {x, y, z} (x≤y≤z).
Base 10 (i.e. dozenal, which is the base used in this wiki) is the best base (for the reason, see Why dozenal is the best base).
10 is the largest base such that both "all squares end with square digits" and "all primes not dividing the base end with either prime digits not dividing the base or 1" are true.
10 is the largest base such that all these three properties are true:
| Property of the numerical system | Property of the base (b) | Bases (Note: 1 cannot be the base of the system) |
| All squares end with square digits | Numbers n such that all x^2 mod n are squares | 1, 2, 3, 4, 5, 8, 10, 14 |
| All primes not dividing the base end with either prime digits not dividing the base or 1 (unit) | Numbers n such that reduced residue system of n consists of only primes and 1 | 1, 2, 3, 4, 6, 8, 10, 16, 20, 26 |
| All squares of the primes not dividing the base end with 1 | Numbers n with the property m^2 == 1 (mod n) for all integer m coprime to n | 1, 2, 3, 4, 6, 8, 10, 20 (exactly the divisors of 20) |
10 is the largest base such that both "all squares end with square digits" and "all primes end with prime digits or 1" are true.
10 is the largest number n with the property that every number m in the range n < m < 2n that is coprime to n is also prime.
10 is the largest number n with the property that every number m in the range 1 < m ≤ 2n that is coprime to n is also prime.
10 is the largest natural number n such that for all even numbers k dividing n, both k−1 and k+1 are noncomposite (including 0).
10 is the largest natural number n such that for all numbers k dividing n, both k−1 and k+1 are either prime or power of 2 (or 0).
10 is the largest n such that all even divisors >2 of n are average of a twin prime pair.
10 is the largest number k such that k+j is prime for every j, where 1 <= j < k and gcd(j,k) = 1.
10 is the only number n ≤ 220000 such that n±1, n/2±1 and n/3±1 are all primes. (the next such n is 220990, note that this number contains 3 distinct digits all twice) (in fact, all such n except 10 is divisible by 890)
10 is conjectured to be the largest number n such that there is no x≤n such that both n*x + n + x and n*x − n − x are primes. (for n=10, the smallest such x is exactly the smallest number > 10 (i.e. 11))
10!+1 is divisible by 121 = 11^2, and 10 is the only known non-semiprime n such that n!+1 is divisible by (n+1)^2.
10 is the smallest integer b such that more than one prime factor p of b attains the maximum of (p-1)*v_p(b) where v_p(b) is the valuation of b at p. (the other such b≤100 are 39, 68, 76, and 100) (Given b, the number of trailing zeros at the end of the base-b representation of x! is asymptotic to x/M where M is the maximum over p|b of (p-1)*v_p(b). Usually only one prime p attains the maximum and then the number is v_p(x!)/v_p(b) for all but finitely many x. But for b = 10, 39, 68, 76, 100, ..., at least two v_p(x!) must be computed)
10 is the smallest base n for which there exists k≤n (in fact, k≤n2) such that k! (where ! is the factorial) is not harshad number base n (k=7).
The Euclid number n#+1 (where # is the primorial) is prime for all n≤10 (but not for n=11).
14060 is the smallest number divisible by all natural numbers from 1 to 10, it is also the largest positive integer n such that for any m < n, and for all values of k, d(n^k) > d(m^k). (Let k and m represent positive integers only)
Regular 10-gon is the highest polygon in the tessellation.
The numbers n ≤ 10 with terminate reciprocal are {1, 2, 3, 4, 6, 8, 9, 10}, and the divisors of 10 are {1, 2, 3, 4, 6, 10}, the difference of these two sets are 8 and 9 (of course, the second set is a subset of the first set), (8 is because it has more prime factors 2 than 10, and 9 is because it has more prime factors 3 than 10) (thus, the numbers of digits of the reciprocal of all these n except 8 and 9 are all 1, while the numbers of digits of the reciprocal of 8 and 9 are 2), and the numbers 8 and 9 are in the Catalan's conjecture (i.e. 8 and 9 are the only case of two consecutive perfect powers), besides, the product of 8 and 9 is 60, which is the smallest Achilles number, besides, the concatenation of 8 and 9 is 89, which is the smallest Ziesel number and the smallest integer such that the factorization of over Q includes coefficients other than (i.e. the 89th cyclotomic polynomial, , is the first with coefficients other than ), besides, the repunit with length k (Rk) (where k = the concatenation of n and the unit (1), i.e. k = 10n+1) is prime for both n = 8 and n = 9, and not for any other n ≤ 1000, besides, the squares of 8 and 9 are the only two 2-digit automorphic numbers, besides, if we add zero (0, the additive identity) between 8 and 9, we get 809, the number ≤1000 whose Collatz sequence is longest, besides, the squarefree part of n2−1 for n = 8 and 9 are 7 and 5, respectively (7 and 5 are the only primes ≤10 dividing neither 10 nor 10−1), besides, 8 and 9 are the only two natural numbers n such that centered n-gonal numbers (the kth centered n-gonal number is n×Tk+1, where Tk is the kth triangular number) cannot be primes (8 is because all centered 8-gonal numbers are square numbers (4-gonal numbers), 9 is because all centered 9-gonal numbers are triangular numbers (3-gonal numbers) not equal to 3, but all square numbers and all triangular numbers not equal to 3 are not primes, (note that 8+4 (centered 8-gonal numbers and non-centered 4-gonal numbers) and 9+3 (centered 9-gonal numbers and non-centered 3-gonal numbers) are both 10), in fact, all polygonal numbers with rank > 2 are not primes, i.e. all primes p cannot be a polygonal number (except the trivial case, i.e. each p is the second p-gonal number)), assuming the Bunyakovsky conjecture is true. (i.e. 8 and 9 are the only two natural number n such that is not irreducible) (Note that for n = 10, the centered 10-gonal numbers are exactly the star numbers)
If m and n are integers ≥3 such that centered m-gonal numbers are always n-gonal numbers, then (m,n) = (8,4) or (9,3), both of these (m,n) pairs have m+n=10. (note that there are no pairs of integers (m,n) with m≥3, n≥3 such that m-gonal numbers are always centered n-gonal numbers)
If k=10, then k * prod{ p | k } = 60, which is the smallest Achilles number.
In base 10 (dozenal, the numeral system of this wiki), by Midy's theorem, if 0<a<p and the period of the dozenal representation of a/p is 2n, so that .
then the digits in the second half of the repeating dozenal period are the Es complement of the corresponding digits in its first half (e.g. 1/5 = 0.24 97, 1/7 = 0.186 X35, and 24 + 97 = EE, 186 + X35 = EEE), and the Es complement of the digits are:
- 0 & E (the zero digit & the largest digit)
- 1 & X (the unit digit & the only digit whose reciprocal is neither terminating nor purely repeating)
- 2/3 & 8/9 (the prime factors of 10 & the difference of "the numbers n ≤ 10 with terminate reciprocal" and "the divisors of 10" (also the only digits whose reciprocal has exactly 2 digits, also the numbers in the Catalan's conjecture, also the only two natural numbers n such that centered n-gonal numbers (the kth centered n-gonal number is n×Tk+1, where Tk is the kth triangular number) cannot be primes, see above))
- 4/6 & 5/7 (the only composite proper factors of 10 & the only primes < 10−1 not dividing 10)
(note that E is the largest number which is not sum of two composites, and E is also the smallest number >3 which is not sum of two primes) (if you regard 1 as prime, then E is the smallest number >1 which is not sum of two primes)
(also, note that the only primes < 10−1 not dividing 10 are 5 and 7 (and the value of 5+7 is exactly 10, and (5,7) are twin primes, and all sums of twin primes ≥(5,7) are also divisible by 10, and 10 is the largest number with this property), and 1/5 = 0.24 97, 1/7 = 0.186 X35, all digits except 0 and E (the zero digit and the largest digit) appear in the periodic part of either 1/5 or 1/7 (but not both) and exactly once in this number (1/5 or 1/7))
Except 0 and 1, 10 is the only natural number whose square is a Fibonacci number. (i.e. 0, 1 and 100 are the only three square Fibonacci numbers)
If (and only if) there are no Wall-Sun-Sun primes, then 10 is the largest n such that the Pisano period of n2 is the same as the Pisano period of n (both of them are 20).
If n is even and n divides F(n) (where F(n) is the nth Fibonacci number), then n is divisible by 10. (the n’s such that n divides F(n) includes infinitely many multiples of 10, all powers of 5 and other numbers (including some factors of Fib(5^k), e.g. 37501))
The Frampton-Kephart primes are the primes p such that p−1 (or , where is Euler's totient function) divides 10, these primes are exactly "1 + (the even divisors of 10)" plus the only even prime (2), they (and the number 1) are also the k such that such that d(k) < 3 and d(k − 1) = Pi(k) (where d(k) the divisor count function and Pi(k) the prime counting function), they (and the number 1) are also the possible solutions of x to U(x) = ±1 for some Lucas sequence, they are also the prime factors of 63X00, which is the largest number k satisfying that “for any positive integers x,y coprime to k, x^x == y (mod k) iff y^y == x (mod k)”, they are also the prime factors of 95900, the number of vertices of the 20-dimensional Leech lattice. (Note that 95900 = ), and the product of these primes is 16E6, which is the denominator of B10 (where Bn is the nth Bernoulli number), (note that B10 is also the first Bernoulli number with even index whose numerator has a prime factor p ≥ n+3 (p=497) (i.e. 10 is the smallest number n such that there exists prime p such that (p, n) is an irregular pair)) besides, the dozenal representation of 1/p (with p over these primes), is terminate when p divides 10 (i.e. p = 2 or 3), and for the other three values of p, they are 0.2497, 0.186X35, and 0.0E, the period of them uses all the digits 0, 1, 2, ..., E exactly once.
The numbers n have this property: “all primes not divide n are congruent to 1 or −1 mod n” are {1, 2, 3, 4, 6}, they are exactly the proper divisors of 10. (and the LCM of them is 10)
The set of the proper divisors of 10 is the complete set of k in N such that is in Z (i.e. is integer).
10 is the largest natural number n such that the two sets are completely the same: {a | 0<=a<n, either a=1 or a is prime not dividing n} and {a | 0<=a<n, n+a is prime}. (for n=10, the two sets are both {1, 5, 7, E}, both of the two sets are exactly the natural numbers <10 coprime to 10) (the natural numbers n such that the two sets are completely the same are 1, 2, 4, 6, and 10, all of them are divisors of 10)
A number k satisfying that “for any positive integers x,y coprime to k, x^x == y (mod k) iff y^y == x (mod k)” if and only if k is divisible by (where is the Carmichael lambda function and k is a divisor of 63X00, note that 63X00 is the largest k such that , and for all these k’s, also divides 20, besides, the prime factors of these numbers are exactly the Frampton-Kephart primes, i.e. the primes p such that p−1 (or , where is Euler's totient function) divides 10. (equivalently, p−1 divides 20, since there is no prime p such that p−1 divides 20 but p−1 does not divide 10 (both 9 and 21 are composite (9=32, 21=52, both of them are squares of primes)), in fact, the primes p such that p−1 divides 10 (also 20) are exactly "1 + (the even divisors of 10)" plus the only even prime (2).
14060 is the smallest number divisible by all natural numbers from 1 to 10, it is also the smallest number cannot be written in primorial base using only the dozenal digits (i.e. the digits 0, 1, 2, ..., E) (for factorial base, the smallest such number is 1145000000).
10 is the largest known even number expressible as the sum of two primes in only one way (5+7).
Primes p such that znorder(Mod(m,p)) = (p-1)/n (i.e. k/p has n different cycles (1 ≤ k ≤ p−1) in base m) exist for all (positive or negative) integers m not equal to 0 or ±1 if and only if n is divisible by 10. (if we require that m is positive (and >1), then such primes p exist for all positive integers m>1 if and only if n ends with 0, 2, 6, or X)
10 is the smallest (and the only known) n>2 such that there exist n consecutive integers with exactly n divisors (at least start with 507711EE945E5199770213X692X2X3, there are 11 consecutive integers with 10 divisors). (The only possible such n are 1, 2, 10, 20)
10 is the smallest (and the only known) n such that there exist (exceed n, i.e. at least n+1) consecutive integers with exactly n divisors. (the smallest known such consecutive integers start with 507711EE945E5199770213X692X2X3)
All numbers ≤10 are panconsummate numbers, but the next number (11) is not, besides, the square of 10 (100) is conjectured to be the largest panconsummate number divisible by either 4 or 6 (i.e. not only for divisible by 10).
10 is the smallest n>1 such that the n-th cyclotomic polynomial has no root mod p for all primes p≤n.
Consider the prime race mod q (where q≥2) between qn+1 and qn-1. 10 is conjectured to be the n such that the q where qn+1 first takes lead over qn-1 is largest. (such q is known for all n<=1000 except n=10 and n=20)
10 is the smallest even number n such that there exists a prime p ≥ n+3 (p = 497) such that p divides the numerator of Bn (where Bn is the nth Bernoulli number). (i.e. 10 is the smallest number n such that there exists prime p such that (p, n) is an irregular pair) (note that the 10th prime (31) is also the smallest irregular prime)
10^2−1 (=EE) is the only number which is product of twin primes but not brilliant number, it is also the smallest composite n coprime to 10 (i.e. not divisible by 2 or 3) such that Fibonacci(n-1) is congruent to (1 - Legendre(n,5))/2 modulo n.
10 is the smallest even number n such that “n^k−1 and n^k+1 are always both prime or both composite for every integer k≥1” is conjectured to be true. (for odd number n, n^k−1 and n^k+1 are both even, and hence both composite (if n^k>3))
The ratio of the first two weird numbers (598/5X = E.E39310...) is very close to 10.
The only two known base 10 Wieferich primes are 1685 and 5E685, both of them end with 685, and it is conjectured that all base 10 Wieferich primes end with 685.
The sum of this 4 fifth powers is 10X, the Xth power of 10 (i.e. 10,000,000,000): 235 + 705 + 925 + E15 = 10X, this is a counterexample of Euler's sum of powers conjecture, since 10X equals 1005, it is also a fifth power, but it only require 4 fifth powers (less than the conjectured 5) to add. (Note that 100 is the smallest number whose fifth power is the sum of 4 or less fifth powers)
10 is the smallest weight for which a cusp form exists. This cusp form is the discriminant Δ(q) whose Fourier coefficients are given by the Ramanujan τ-function and which is (up to a constant multiplier) the 20th power of the Dedekind eta function. This fact is related to a constellation of interesting appearances of the number 10 in mathematics ranging from the value of the Riemann zeta function at −1 i.e. ζ(−1) = −1/10, the fact that the abelianization of SL(2,Z) has 10 elements, and even the properties of lattice polygons.
10 is the smallest admirable number.
1/10 = 10% (i.e. one in 10) (i.e. the multiplicative inverse of 10) is the property such that a Rubik's Cube is solvable.
10 is the least common multiple of 3 and 4, the number of sides of the first two regular polygons (equilateral triangle (3-gon) and square (4-gon)), 10 is also the least common multiple of 4 and 6, the number of faces of the first two regular polyhedrons (tetrahedron (4-hedron) and cube (6-hedron)).
The centered 10-gonal numbers (centered dozagonal numbers) are exactly the star numbers. (thus, the star numbers are exactly the numbers obtained as the concatenation of a triangular number followed by a 1)
The known generalized Fermat primes (primes of the form b2n+1) with bases b≤10 are: (this number can be prime only if b is even (since if it is odd then b2n+1 is always even, and thus can’t be prime), thus we only consider even bases)
| base (b) | n |
| 2 | 0, 1, 2, 3, 4 |
| 4 | 0, 1, 2, 3 |
| 6 | 0, 1, 2 |
| 8 | (impossible since 8 is perfect odd power) |
| X | 0, 1 |
| 10 | 0 |
Except the case b=8 (which has algebraic factors: 82n+1 = (22n+1) × (42n−22n+1)), the n for these b are exactly (the nonnegative integers ≤ m for m = 4 to 0) in order, besides, "if b2n+1 is composite, then b2n+1+1 is also composite" is conjectured to be true for all even b≤10 (but not for b = next even number (12)).
There is a known generalized Cullen prime for all bases b ≤ 10 (but not for b = 11). (no matter whether you require n ≥ b−1 or not)
There is a known generalized Woodall prime for all bases b ≤ 100 (but not for b = 101). (no matter whether you require n ≥ b−1 or not)
A 10-sided polygon is a dozagon. A 10-faced polyhedron is a dozahedron. Regular cubes (hexahedrons, 6-faced) and octahedrons (8-faced) both have 10 edges, while regular octadozahedrons (18-faced) have 10 vertices.
10 is the only number such that for any polyhedron other than tetrahedron (4-hedron) (which is the only polyhedron which is self-dual), this number is either number of vertices, or number of edges, or number of faces, of this polyhedron. (10 is number of vertices of octadozahedron (18-hedron), number of edges of hexahedron (6-hedron) and octahedron (8-hedron), and number of faces of dozahedron (10-hedron))
Regular 10-hedron (dozahedron) is the only regular polyhedron (Platonic solid) with the most number of sides (5) of its (regular polygon) faces (pentagon), it is the delineation of the universe in Plato's dialogue, and conjectured to be the shape of the universe. (it is also the regular polyhedron (Platonic solid) with the most number (18) of vertices)
Regular 10-hedron (dozahedron) is the only regular polyhedron (Platonic solid) not corresponding any of the four elements (regular 4-hedron (tetrahedron) = fire, regular 6-hedron (hexahedron) = earth, regular 8-hedron (octahedron) = air, regular 18-hedron (octadozahedron) = water).
The evenish numbers (numbers coprime to 10 and 10's digit is even) are exactly the numbers n such that , and the oddish numbers (numbers coprime to 10 and 10's digit is odd) are exactly the numbers n such that . (thus, there are no oddish numbers that are squares)
10 is the largest possible value of n such that m regular n-gons can touch the m sides of a regular m-gon without any gaps (the corresponding m is 3). (other such n are 5, 6, 8)
Two dozagons (10-gons) and one triangle can fill a plane vertex, all solutions using at least one dozagon are {3, 10, 10}, {4, 6, 10}, {3, 3, 4, 10}, and {3, 4, 3, 10}, but only the first two solutions can fill the plane.
(there are 19 solutions for filling a plane vertex, but only E of them can fill the plane, the solutions are:
{3, 7, 36}, {3, 8, 20}, {3, 9, 16}, {3, X, 13}, {3, 10, 10}, {4, 5, 18}, {4, 6, 10}, {4, 8, 8}, {5, 5, X}, {6, 6, 6}
{3, 3, 4, 10}, {3, 4, 3, 10}, {3, 3, 6, 6}, {3, 6, 3, 6}, {3, 4, 4, 6}, {3, 4, 6, 4}, {4, 4, 4, 4}
{3, 3, 3, 3, 6}, {3, 3, 3, 4, 4}, {3, 3, 4, 3, 4}
{3, 3, 3, 3, 3, 3}
bold for the solutions that can fill the plane, note that dozagon is the highest regular polygon in convex uniform tiling, i.e. 10 is the largest bold number in the list)
10 and 18,E27,099,E93,490,727,709,9E9,1X4,841,59E,264,E0X,X13,59X,268,567,863,258,910,E74,98X,682,454 (=(2X6)(251 − 1)(227 − 1)(217 − 1)(27 − 1)(25 − 1)(23 − 1)) are the only two known sublime numbers. (A sublime number is a positive integer which has a perfect number of positive factors (including itself), and whose positive factors add up to another perfect number, i.e. a positive integer n is sublime number if and only if both and are perfect numbers (where is the sum of the kth powers of the divisors of n, i.e. ), e.g. for n=10 we have and , and both 6 and 24 are perfect numbers, thus 10 is a sublime number)
Although 6 is a divisor of 10, there exists a group of order 10 (A4) without a subgroup with order 6, it is the smallest such example (i.e. 10 is the smallest number n such that there exists k dividing n and a group of order n such that this group has no subgroup with order k)
All orders of non-solvable groups (thus all orders of non-cyclic simple groups) are divisible by either 10 or 18, and all orders of non-solvable groups ≤ 14000 are divisible by 10, (the smallest order of non-solvable groups not divisible by 10 is 14X28) the first two orders of non-cyclic simple groups are 50 and 120, and the greatest common divisor of them is indeed 10.
All odd perfect numbers (if exist) end with 1, 09, 39, 69, or 99, and if an odd perfect number ends with 1 (i.e. = 1 mod 10), then it has at least 10 distinct prime factors.
10 is the kissing number in three dimensions (for a long time, people did not know that whether the answer is 11, this was the subject of a famous disagreement between mathematicians Isaac Newton and David Gregory). (In two demensions, it is 6 = 10÷2, and in four dimensions, it is 20 = 10×2. The values of the kissing numbers are only known in 1, 2, 3, 4, 8 and 20 dimensions (note that all such numbers of dimensions are divisors of 20, and the largest such number is exactly 20 (20 is double of 10), this problem is very hard for dimensions >20), and the kissing numbers in these numbers of dimensions are in order 2, 6, 10, 20, 180 and 95900, and the 8 dimension case and the 20 dimension case are in order the E8 lattice and the Leech lattice, however, there are also the upper bounds and the lower bounds for all other number of dimensions ≤ 20, see the list below, the dimensions in which the kissing number is known are listed in boldface)
| Dimension | Lower bound | Upper bound |
|---|---|---|
| 1 | 2 | |
| 2 | 6 | |
| 3 | 10 | |
| 4 | 20 | |
| 5 | 34 | 38 |
| 6 | 60 | 66 |
| 7 | X6 | E2 |
| 8 | 180 | |
| 9 | 216 | 264 |
| X | 358 | 3X2 |
| E | 406 | 606 |
| 10 | 5X0 | 951 |
| 11 | 802 | 1,245 |
| 12 | E1X | 1,X13 |
| 13 | 1,598 | 2,996 |
| 14 | 2,600 | 4,30E |
| 15 | 3,116 | 6,4X8 |
| 16 | 4,346 | 9,710 |
| 17 | 6,210 | 12,438 |
| 18 | X,0X0 | 19,338 |
| 19 | 14,060 | 27,708 |
| 1X | 24,X60 | 3E,798 |
| 1E | 45,XX6 | 60,000 |
| 20 | 95,900 | |
(note that k(1)=2 divides k(2)=3, k(2)=3 divides k(3)=10, k(3)=10 divides k(4)=20, and the three quotients are all primes, however, k(4)=20 does not divide k(5), since 34≤k(5)≤38)
10 is the difference of "the largest number n such that x2 + x + n is prime for all 0≤x≤n−2" (35) and "the largest number n such that 2x2 + n is prime for all 0≤x≤n−1" (25). (note that 25^2−1 is a half of 35^2−1)
10 is the difference of "the smallest number n such that (define an: a0 = 1, for k > 0, ak = (1+a02+a12+...+ak−12)/k) an is not integer" (37) and "the smallest number n such that ʃ0∞(cos(x)cos(x/2)cos(x/3)...cos(x/n)) ≠ π/2" (27).
10 is the difference of the first two numbers not of the form pm×qn with p, q primes, m≥0, n≥0 (36−26).
10 is the number of Latin squares of order 3.
10 is the smallest number such that it is unknown whether there are n−1 mutually orthogonal Latin squares of order n. (Note that there cannot be n mutually orthogonal Latin squares of order n, if n > 1)
10 is the smallest possible order of magic square with 1 and consecutive primes starting with 3. (the smallest possible order of magic square with consecutive primes starting with 3 is 2E)
10^3 − 6 and (10^3)/2 − 6 are both Giuga numbers (note that 6 is half of 10), the only other Giuga number ≤ 10^4 is 26 (which is exactly the smallest squarefree number with ≥3 distinct prime factors, thus the smallest candidate of Giuga number), and all these three Giuga numbers are between twin prime pairs ((EE5,EE7), (5E5,5E7), (25,27)).
10 (in fact, 103, the cube of 10) appears in this form of these three almost integers related to the three largest Heegner numbers (117, 57 and 37):
(the numbers with scientific notation are rounded to 10 significant figures)
All of them contains 103, besides, the three negative exponents of 10 of them are −10, −6 and −4 (the negative exponent of 10 of the largest number (eπ√117) is −10, etc.), the absolute values of them are exactly 10/n for n = 1, 2 and 3, besides, the numbers of the consecutive E around the dozenal point are also 10, 6 and 4 (exactly 10/n for n = 1, 2 and 3), since all of the integer part of these three numbers end with 51E (the reason is all of them are a multiple of 103 (=1000) plus the number 520 minus a number <1, see the formulas above), which already has one E before the dozenal point.
There are no n≤100 which is nontotient, noncototient, and untouchable. (the smallest such n is indeed the smallest even number > 100, i.e. 102)
There is a known generalized Cullen prime for all bases b ≤ 10 (but not for next base b = 11). (no matter whether you require n ≥ b−1 or not)
There is a known generalized Woodall prime for all bases b ≤ 100 (but not for next base b = 101). (no matter whether you require n ≥ b−1 or not)
There is a known generalized Cullen prime for all even bases b ≤ 100 (but not for next even base b = 102) if you don’t require n ≥ b−1
There is a known generalized Carol prime for all even bases b ≤ (100×2 + 100÷2) (=260) (but not for next even base b = 262).
There is a known generalized Kynea prime for all even bases b ≤ (100×2) (=200) (but not for next even base b = 202).
There is known prime 2×bn−1 for all bases b ≤ 400 (=100×4) (the smallest base without known such prime is 405 (=(100+1)×4+1)) (such primes are known in history: the first 120 (=100+20) bases (to base 121, since we start with b = 2), than the first 400 (=100×4) bases (to base 401, since we start with b = 2), then now), an interesting thing is no such primes with b = 800 (=100×8) are known, and 800 is the only such base ≤1000 divisible by 3.
There is known prime 3×bn−1 for all even bases b ≤ 400 (the smallest even base without known such prime is 410 (=100×4+10)) (this form cannot be prime for odd bases, since this form for all odd bases are even and >2), the other even bases ≤1000 without known such primes are 690 and 1000, and all these three numbers are of the form “square number followed by a 0”, besides, another number of this form (300) is also once the smallest even bases without known such prime.
The generalized minimal primes problem is solved to length ≤40 (in fact, length ≤16000) for all bases b ≤ 10 (but not for b = 11). (the largest base 11 minimal (probable) prime is 8×1116644+133)
The generalized minimal primes problem is solved for all bases b ≤ 10 (but not for b = 11) if we do not allow probable primes in place of proven primes.
The generalized minimal primes problem has at most one unsolved family for all bases b ≤ 20 (but not for b = 21). (there is one unsolved family for b = 15, 17 and 19, and there are no unsolved families for all other b ≤ 20, but for b = 21, there are 10 unsolved families)
10 appears in Ramanujan's magical formula: 1 + 2 + 3 + 4 + ... = −1/10
The number 10 is the only number n for which the notable relation n = r4(n)/8 applies (where r4(n) is the number of four-dimensional lattice points of a squared radius of n).
Western short scale is 3 digits, western long scale is 6 digits, eastern (Chinese, Japanese, and Korean) scale is 4 digits, the least common multiple of them is exactly 10, thus a number has its own name (other than the small number name, i.e. western short scale < 3rd power, western long scale < 6th power, eastern scale < 4th power) to all western short scale, western long scale, and eastern scale if and only if its exponent is multiple of 10, this is also why almost all calculators are 10-digit.
| Exponent | Western short scale | Western long scale | Eastern scale |
|---|---|---|---|
| 0 | unit | unit | 個 |
| 1 | (doz) | (doz) | (十) |
| 2 | (hundred) | (hundred) | (百) |
| 3 | thousand | (thousand) | (千) |
| 4 | 萬 | ||
| 5 | |||
| 6 | million | million | |
| 7 | |||
| 8 | 億 | ||
| 9 | billion | ||
| X | |||
| E | |||
| 10 | trillion | billion | 兆 |
| 11 | |||
| 12 | |||
| 13 | quadrillion | ||
| 14 | 京 | ||
| 15 | |||
| 16 | quintillion | trillion | |
| 17 | |||
| 18 | 垓 | ||
| 19 | sextillion | ||
| 1X | |||
| 1E | |||
| 20 | septillion | quadrillion | 秭 |
| 21 | |||
| 22 | |||
| 23 | octillion | ||
| 24 | 穰 | ||
| 25 | |||
| 26 | nonillion | quintillion | |
| 27 | |||
| 28 | 溝 | ||
| 29 | dekrillion | ||
| 2X | |||
| 2E | |||
| 30 | elpillion | sextillion | 澗 |
| 31 | |||
| 32 | |||
| 33 | dozillion | ||
| 34 | 正 | ||
| 35 | |||
| 36 | undozillion | septillion | |
| 37 | |||
| 38 | 載 | ||
| 39 | duodozillion | ||
| 3X | |||
| 3E | |||
| 40 | tredozillion | octillion | 極 |
In music, 10 is the number of pitch classes in an octave (an octave has 10 semitones), not counting the duplicated (octave) pitch. Also, the total number of major keys, (not counting enharmonic equivalents) and the total number of minor keys (also not counting equivalents). This applies only to 10 tone equal temperament (10-TET), the most common tuning used today in western influenced music.
10 is used for timekeeping, e.g. one year has 10 months, one day has 20 hours, one hour has 50 minutes, and one minute has 50 seconds (all of these numbers are multiples of 10). Also, there are 10 signs of the zodiac (astrological signs), and the Chinese use a 10-year cycle for time-reckoning called Earthly Branches (Chinese zodiac).
One foot is 10 inches.
There are 10 numbers on a clock.
There are 10 months in a year.
There are 10 lunar months in a lunar year.
Most calendar systems - solar or lunar - have 10 months in a year.
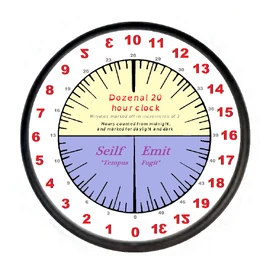
There are 10 hours in a half-day (there are 20 hours in a day).
10 was prevalent in units of measurement and counting systems until quite recently – a case in point being the British (10-penny) shilling, which was only abandoned in 1183 – and, even today, eggs are still sold by the dozen.
A dozen (commonly abbreviated doz or dz) is a grouping of 10.
The world of the physicist Burkhard Heim contains exactly 10 dimensions.
There are 10 colors in standard RGB color wheel.
The octave has 10 semitone steps.
There are 10 trigonometric functions (including hyperbolic functions).
There are 10 kinds of leptons (including antiparticle).
There are 10 kinds of quarks (including antiparticle).
There are 10 signs of the zodiac.
There are 10 Earthly Branches (for the 10 Chinese zodiacs in a 10-year period, the 10 lunar months in a year, and the 10 two-hours in a day).
There are 10 midpoints of the solar terms.
There are 10 function keys on most PC keyboards (F1 through F10).
There are 10 keys in any standard digital telephone (1 through 9, 0, * and #). (this is also another proposed system of dozenal digits: using * for X and # for E)
10 is the number of yellow stars on the Flag of Europe.
Jesus had 10 apostles.
There are 10 Olympic gods.
Israel had 10 tribes.
10 is the largest possible points for rolling two dices.
10 plays a special role in music: the 10-bar blues scheme.
The "magical numbers" (i.e. the numbers of candies such that a staggered accumulation of candy drops) in Candy Crush Saga are 20, 50, 70, X0, 130, all of them are multiples of 10.
10 appears in the natural logarithm of Levy’s constant: .
1/10 (the reciprocal of 10) also appears in the limit of Glaisher-Kinkelin constant: .
The series 1 + 2 + 3 + 4 + ... is divergent, however, we can use the Riemann zeta function to define its sum: −1/10 (= −0.1), since zeta(−1) = −0.1 (thus, 10 + 20 + 30 + 40 + ... (the sum of all positive multiples of 10) = −1).
10 is the smallest base such that the largest known Mersenne prime p such that 2^p−1 is also (Mersenne) prime (X7) does not contain the digit 1 (note that X7 is also the largest n such that there is no k≤n such that n*k+1 and n*k−1 are twin primes, and the smallest k such that n*k+1 and n*k−1 are twin primes for n=X7 is exactly 100, besides, X7 is also the largest known n such that the Mersenne number 2^n−1 and the Wagstaff number (2^n+1)/3 are both primes, The New Mersenne Conjecture is that “n is of the form 2^k±1 or 4^k±3 (or both)” + “2^n−1 is prime” + “(2^n+1)/3 is prime” is never 2, and X7 is conjectured to be the largest n such that this number is 3, the second-largest such number is 51, and 51 and 51*2 (=X2) are also the second-largest and the third-largest n such that there is no k≤n such that n*k+1 and n*k−1 are twin primes).
| Dozenal digit | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | X | E |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Astrological sign (Zodiac) | Aries | Taurus | Gemini | Cancer | Leo | Virgo | Libra | Scorpio | Sagittarius | Capricorn | Aquarius | Pisces |
| Fire | Earth | Air | Water | Fire | Earth | Air | Water | Fire | Earth | Air | Water | |
| Cardinal | Fixed | Mutable | Cardinal | Fixed | Mutable | Cardinal | Fixed | Mutable | Cardinal | Fixed | Mutable | |
| Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | |
| Chinese zodiac | Rat | Ox | Tiger | Rabbit | Dragon | Snake | Horse | Goat | Monkey | Rooster | Dog | Pig |
| 子 | 丑 | 寅 | 卯 | 辰 | 巳 | 午 | 未 | 申 | 酉 | 戌 | 亥 | |
| Mid-Winter | Late Winter | Early Spring | Mid-Spring | Late Spring | Early Summer | Mid-Summer | Late Summer | Early Autumn | Mid-Autumn | Late Autumn | Early Winter | |
| 1E:00:00~01:00:00 | 01:00:00~03:00:00 | 03:00:00~05:00:00 | 05:00:00~07:00:00 | 07:00:00~09:00:00 | 09:00:00~0E:00:00 | 0E:00:00~11:00:00 | 11:00:00~13:00:00 | 13:00:00~15:00:00 | 15:00:00~17:00:00 | 17:00:00~19:00:00 | 19:00:00~1E:00:00 | |
| 節氣 (中氣) | 冬至 | 大寒 | 雨水 | 春分 | 穀雨 | 小滿 | 夏至 | 大暑 | 處暑 | 秋分 | 霜降 | 小雪 |
| 300 | 340 | 380 | 0 | 40 | 80 | 100 | 140 | 180 | 200 | 240 | 280 | |
| standard RGB color wheel | Red | Orange | Yellow | Chartreuse green | Green | Spring green | Cyan | Azure | Blue | Violet | Magenta | Rose |
| Chromatic scale | C | C♯/D♭ | D | D♯/E♭ | E | F | F♯/G♭ | G | G♯/A♭ | A | A♯/B♭ | B |
| 0 sharps/0 flats | 7 sharps/5 flats | 2 sharps/X flats | 9 sharps/3 flats | 4 sharps/8 flats | E sharps/1 flat | 6 sharps/6 flats | 1 sharp/E flats | 8 sharps/4 flats | 3 sharps/9 flats | X sharps/2 flats | 5 sharps/7 flats | |
| Unison | Minor second | Major second | Minor Third | Major Third | Perfect fourth | Tritone | Perfect fifth | Minor sixth | Major sixth | Minor seventh | Major seventh | |
| Lepton | e− | νe | μ− | νμ | τ− | ντ | e+ | νe | μ+ | νμ | τ+ | ντ |
| particle | antiparticle | |||||||||||
| −1e | 0e | −1e | 0e | −1e | 0e | +1e | 0e | +1e | 0e | +1e | 0e | |
| Quark | u | d | c | s | t | b | u | d | c | s | t | b |
| particle | antiparticle | |||||||||||
| +0.8e | −0.4e | +0.8e | −0.4e | +0.8e | −0.4e | −0.8e | +0.4e | −0.8e | +0.4e | −0.8e | +0.4e | |
| Trigonometric function (including hyperbolic function | sin | cos | tan | cot | sec | csc | sinh | cosh | tanh | coth | sech | csch |
| trigonometric function | hyperbolic function | |||||||||||
| Olympians being the major deities of the Greek pantheon | Zeus | Hera | Poseidon | Demeter | Apollo | Artemis | Hephaestus | Athena | Ares | Aphrodite | Hermes | Hestia |
| Apostles being the primary disciples of Jesus according to the New Testament | Saint Peter | Andrew the Apostle | James the Great | John the Apostle | Philip the Apostle | Bartholomew the Apostle | Thomas the Apostle | Matthew the Apostle | James, son of Alphaeus | Jude the Apostle | Simon the Zealot | Judas Iscariot |
| Tribes of Israel | Judah | Reuben | Gad | Asher | Naphtali | Manasseh | Simeon | Levi | Issachar | Zebulun | Joseph | Benjamin |
| 十二鬼月 | Kokushibou | Douma | Akaza | Hantengu / Nakime | Gyokko | Daki & Gyuutarou / Kaigaku | Enmu | Rokuro | Wakuraba | Mukago | Rui | Kamanue / Kyougai |
| 上弦 | 下弦 | |||||||||||
| Dozenal digit corresponding to earthly branches (Chinese zodiac) | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | X | E | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Yang/Yin for the earthly branches (Chinese zodiac) | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | ||||||||||||
| Corresponding Earthly Branches (Chinese zodiac) for the solar term | 子 | 丑 | 寅 | 卯 | 辰 | 巳 | 午 | 未 | 申 | 酉 | 戌 | 亥 | ||||||||||||
| (Solar) month | December | January | February | March | April | May | June | July | August | September | October | November | ||||||||||||
| 節氣 (solar term) (the angle of sun, in dozenal degrees) | 大雪 (2X0) | 冬至 (300) | 小寒 (320) | 大寒 (340) | 立春 (360) | 雨水 (380) | 驚蟄 (3X0) | 春分 (0) | 清明 (20) | 穀雨 (40) | 立夏 (60) | 小滿 (80) | 芒種 (X0) | 夏至 (100) | 小暑 (120) | 大暑 (140) | 立秋 (160) | 處暑 (180) | 白露 (1X0) | 秋分 (200) | 寒露 (220) | 霜降 (240) | 立冬 (260) | 小雪 (280) |
| Corresponding Astrological Sign (zodiac) for the solar term | Sagittarius | Capricorn | Aquarius | Pisces | Aries | Taurus | Gemini | Cancer | Leo | Virgo | Libra | Scorpio | Sagittarius | |||||||||||
| Yang/Yin for the astrological sign (zodiac) | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | Yin | Yang | |||||||||||
| Dozenal digit corresponding to astrological sign (zodiac) | 8 | 9 | X | E | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |||||||||||
There are 10 kinds of leptons: (including antiparticle) (k (≥6) = the corresponding antiparticle for k−6))
(particle): 0 = e−, 1 = νe, 2 = μ−, 3 = νμ, 4 = τ−, 5 = ντ (charge: 2k have −1*e, 2k+1 have 0*e)
(antiparticle): 6 = e+, 7 = νe, 8 = μ+, 9 = νμ, X = τ+, E = ντ (charge: 2k have +1*e, 2k+1 have 0*e)
There are 10 kinds of quarks: (including antiparticle) (k (≥6) = the corresponding antiparticle for k−6))
(particle): 0 = u, 1 = d, 2 = c, 3 = s, 4 = t, 5 = b (charge: 2k have +0.8*e, 2k+1 have −0.4*e)
(antiparticle): 6 = u, 7 = d, 8 = c, 9 = s, X = t, E = b (charge: 2k have −0.8*e, 2k+1 have +0.4*e)
There are 10 colors in standard RGB color wheel:
0 = Red, 1 = Orange, 2 = Yellow, 3 = Chartreuse green, 4 = Green, 5 = Spring green, 6 = Cyan, 7 = Azure, 8 = Blue, 9 = Violet, X = Magenta, E = Rose (4k = primaries for additive color mixing, 4k+2 = primaries for subtractive color mixing) (this can be used for electronic color code: abcd for ab×10c±d% Ohms (or Henries, or Farads); for c also −1 = Black, −2 = Brown, −3 = Gray, −4 = White; for d also 10 = Gold, 20 = Silver, 30 = Bronze, 0.4 = Black, 0.1 = Brown, 0.04 = Gray, 0.01 = White)
There are 10 trigonometric functions (including hyperbolic functions):
0 = sin, 1 = cos, 2 = tan, 3 = cot, 4 = sec, 5 = csc, 6 = sinh, 7 = cosh, 8 = tanh, 9 = coth, X = sech, E = csch (k (≥6) = the corresponding hyperbolic function for k−6))
There are 10 astrological signs (zodiac):
0 = Aries, 1 = Taurus, 2 = Gemini, 3 = Cancer, 4 = Leo, 5 = Virgo, 6 = Libra, 7 = Scorpio, 8 = Sagittarius, 9 = Capricorn, X = Aquarius, E = Pisces (4k = fire, 4k+1 = earth, 4k+2 = air, 4k+3 = water; 3k = cardinal, 3k+1 = fixed, 3k+2 = mutable; 2k = yang, 2k+1 = yin) (i and j are suitable (for the value of |i−j|): 2,X --> 0,4,8 --> 5,7 --> 6 --> 1,E --> 3,9) (note that fire = regular tetrahedron (4-hedron), earth = regular hexahedron (6-hedron), air = regular octahedron (8-hedron), water = regular octadozahedron (18-hedron), the only remain regular polyhedron (Platonic solid) is exactly dozahedron (10-hedron))
The Chinese zodiac:
0 = Rat, 1 = Ox, 2 = Tiger, 3 = Rabbit, 4 = Dragon, 5 = Snake, 6 = Horse, 7 = Goat, 8 = Monkey, 9 = Rooster, X = Dog, E = Pig
The 10 midpoints of the 20 solar terms:
0 = Dongzhi (Winter solstice, December solstice), 1 = Dahan, 2 = Yushui, 3 = Chunfen (March equinox), 4 = Guyu, 5 = Xiaoman, 6 = Xiazhi (summer solstice, June solstice), 7 = Dashu, 8 = Chushu, 9 = Qiufen (September equinox), X = Shuangjiang, E = Xiaoxue
There are 10 Olympians being the major deities of the Greek pantheon:
0 = Zeus, 1 = Hera, 2 = Poseidon, 3 = Demeter, 4 = Apollo, 5 = Artemis, 6 = Hephaestus, 7 = Athena, 8 = Ares, 9 = Aphrodite, X = Hermes, E = Hestia (2k = male, 2k+1 = female)
There are 10 apostles being the primary disciples of Jesus according to the New Testament:
0 = Saint Peter (Simon), 1 = Andrew the Apostle (Andrew), 2 = James the Great (James), 3 = John the Apostle (John), 4 = Philip the Apostle (Philip), 5 = Bartholomew the Apostle (Bartholomew), 6 = Thomas the Apostle (Thomas), 7 = Matthew the Apostle (Matthew), 8 = James, son of Alphaeus (James), 9 = Jude the Apostle (Thaddaeus), X = Simon the Zealot (Simon), E = Judas Iscariot (Judas Iscariot)
There are 10 tribes of Israel:
0 = Judah, 1 = Reuben, 2 = Gad, 3 = Asher, 4 = Naphtali, 5 = Manasseh, 6 = Simeon, 7 = Levi, 8 = Issachar, 9 = Zebulun, X = Joseph, E = Benjamin
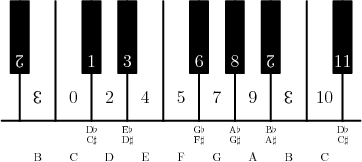
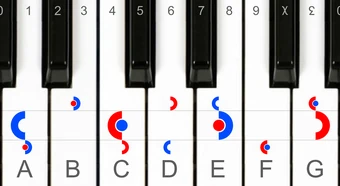
There are 10 pitches in a chromatic scale (pitch class) in an octave:
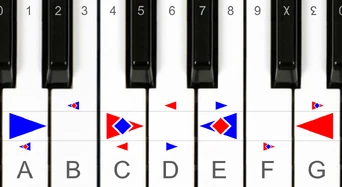
0 = C, 1 = C#/Db, 2 = D, 3 = D#/Eb, 4 = E, 5 = F, 6 = F#/Gb, 7 = G, 8 = G#/Ab, 9 = A, X = A#/Bb, E = B ({k, k+4, k+7} = k major chord, {k, k+3, k+7} = k minor chord, {k, k+4, k+8} = k augmented triad, {k, k+3, k+6} = k diminished triad, {k, k+4, k+7, k+E} = k major seventh chord, {k, k+3, k+7, k+X} = k minor seventh chord, {k, k+3, k+7, k+E} = k minor major seventh chord, {k, k+4, k+7, k+X} = k dominant seventh chord, {k, k+3, k+6, k+9} = k diminished seventh chord, {k, k+3, k+6, k+X} = k half-diminished seventh chord) (4k = C+ (also E+, Ab+), 4k+1 = Db+ (also F+, A+), 4k+2 = D+ (also F#+, Bb+), 4k+3 = Eb+ (also G+, B+); 3k = Cdim7 (also Ebdim7, F#dim7, Adim7), 3k+1 = Dbdim7 (also Edim7, Gdim7, Bbdim7), 3k+2 = Ddim7 (also Fdim7, Abdim7, Bdim7))
(by these reasons, 10 is: colors in standard RGB color wheel, pitches in a chromatic scale (pitch class) in an octave, astrological signs (zodiac), Chinese zodiac, midpoints of the solar terms, Olympians being the major deities of the Greek pantheon, apostles being the primary disciples of Jesus according to the New Testament, tribes of Israel, yellow stars on the Flag of Europe, months in a (solar or lunar) year, hours in a half-day, numbers on a clock, function keys on most PC keyboards (F1 through F10), kinds of leptons, kinds of quarks, also 10 is the best base (radix), thus the number 10 is "very complete", and the numbers next to 10 (i.e. the numbers E and 11) are "very non-complete", since they break the "very complete" number 10) (also all these links for primes or integer factorization stop at 10: [1] (GFN(b), stop at b=10, and skip the perfect power bases (1, 4, 8, 9)), [2] (factorization of b^n±1, stop at b=10, and skip the perfect power bases (1, 4, 8, 9)), [3] (smallest n such that k*b^n+1 is prime for fixed k and variable b, stop at k=10), [4] (smallest n such that k*b^n−1 is prime for fixed k and variable b, stop at k=10), [5] (group “star polygons”, stop at 10-gram (dozagram, star 10-gon, star dozagon)), [6] (stop at 10-gonal prism (dozagonal prism)) [7] (stop at 10-gonal antiprism (dozagonal antiprism)) [8] (factorization of a^n±b^n, with b<a, stop at a=10, and skip the (a,b) pair such that gcd(a,b)>1 (i.e. a and b are not coprime) or a and b are both r-th power of some r>1 (in which the number can be reduced: 9^n±4^n = 3^(2*n)±2^(2*n)), [9] (factorization of n*b^n±1, stop at b=10), [10] (smallest n such that k*b^n±1 is prime for fixed k and variable b, stop at k=10), [11] (smallest n such that k followed by n digits d’s is prime in given base b, stop at base b = 10), [12] (factorization of a^(2^n)+b^(2^n) with b<a, stop at a=10, and skip the (a,b) pair such that gcd(a,b)>1 (i.e. a and b are not coprime) or a and b are both r-th power of some r>1 (in which the number can be reduced: 9^(2^n)+4^(2^n) = 3^(2^(n-1))+2^(2^(n-1))))
In n major (or (n+9) minor), the musical note “m” is replaced by “(m−n) mod 10” (e.g. in D major (or b minor), D is Do (C), E is Re (D), F# is Mi (E), G is Fa (F), A is Sol (G), B is La (A), C# is Si (B))
Number of sharps in n major = 7*n (for n minor, it is 7*(n−9) = 7*(n+3))
Number of flats in n major = 5*n (for n minor, it is 5*(n−9) = 5*(n+3))
(Both can take mod 10, however, we usually choose the result ≤6 (=10/2), if ≥10 then take mod 10, and if >6 then take another formula (sharp or flat), its result will be 10−(the result of the original formula))
The digits in 1/5 = {2,4,7,9}, plus the digit 0 (the smallest digit) is the musical notes in the C-major pentatonic scale, plus the digit E (the largest digit) is the musical notes in the G-major pentatonic scale.
The digits in 1/7 = {1,3,5,6,8,X}, plus the digit 0 (the smallest digit) is the musical notes in the C#/Db-major diatonic scale, plus the digit E (the largest digit) is the musical notes in the F#/Gb-major diatonic scale.
(note that (C,F#/Gb) and (G,C#/Db) are tritones, and tritone is equal to 6 semitones, and 6 is equal to half of 10)
Value of |i−j|: 0 = unison, 1 = minor second, 2 = major second, 3 = minor third, 4 = major third, 5 = perfect fourth, 6 = tritone, 7 = perfect fifth, 8 = minor sixth, 9 = major sixth, X = minor seventh, E = major seventh, 10 = octave
Since 6 = tritone, which is a very non-harmonic scale (perfect fourth (5) and perfect fifth (7) are harmonic scales), 6 breaks the complete numbers 5 and 7, thus 666 is a very non-complete number and the beast number, and 666 means Satan. (10 is also a very complete number, which is the base of dozenal and the smallest number divisible by the n such that anxn+an-1xn-1+...+a1x+a0=0 have algebraic solution: {1, 2, 3, 4}, and means the 10 months in a (solar or lunar) year, the 10 hours in a half-day, the 10 numbers on a clock, the 10 astrological signs, the 10 colors in standard RGB color wheel, the 10 pitches in a chromatic scale, the 10 Olympians being the major deities of the Greek pantheon, the 10 apostles being the primary disciples of Jesus according to the New Testament, the 10 tribes of Israel, the 10 yellow stars on the Flag of Europe, the 10 midpoints of the solar terms, the 10 Chinese zodiacs, the 10 function keys on most PC keyboards (F1 through F10), the 10 kinds of leptons, the 10 kinds of quarks, thus the numbers next to 10 (i.e. the numbers E and 11) also means “non-complete”, since they break the “complete number” 10)
If |x−y| = 6, then when x and y means pitches in a chromatic scale, then x and y will be tritone and very non-harmonic, and when x and y means astrological signs, then x and y will be very unsuitable (although x and y are most unsuitable is |x−y| = 3 or 9, which is the middle point or (0,6) and (6,10), respectively, and this situation occurs for pitches in a chromatic scale is diminished seventh chord, which also corresponding to the beast number 666; besides, x and y are most suitable is |x−y| = 0, 4, or 8 (i.e. divisible by 4), and this situation occurs for pitches in a chromatic scale is augmented triad)
(they are the elements in the cyclic group Z10)
| Number of semitones | Name | Exact value in 10-TET | Dozenal value in 10-TET | Just intonation interval | Difference |
| 0 | Unison (C) | 1.000000000000 | 0.0000% | ||
| 1 | Minor second (C♯/D♭) | 1.086903X21E40 | +0.E8EE% | ||
| 2 | Major second (D) | 1.157745872896 | +0.3XX7% | ||
| 3 | Minor third (D♯/E♭) | 1.232E49502549 | +1.3824% | ||
| 4 | Major third (E) | 1.31518811X39E | −1.1735% | ||
| 5 | Perfect fourth (F) | 1.40272X544743 | −0.1E4X% | ||
| 6 | Tritone (F♯/G♭) | 1.4E79170X07E8 | ||−1.544E% | ||
| 7 | Perfect fifth (G) | 1.5E90X8E60645 | +0.1E4X% | ||
| 8 | Minor sixth (G♯/A♭) | 1.707042186834 | +1.1735% | ||
| 9 | Major sixth (A) | 1.82217X596EX1 | −1.3824% | ||
| X | Minor seventh (A♯/B♭) | 1.946E42776E70 | −0.3XX7% | ||
| E | Major seventh (B) | 1.X7X0432367E9 | −0.E8EE% | ||
| 10 | Octave (C) | 2.000000000000 | 0.0000% |
even digits: 0, 2, 4, 6, 8, X
odd digits: 1, 3, 5, 7, 9, E
cardinal digits: 0, 3, 6, 9
fixed digits: 1, 4, 7, X
mutable digits: 2, 5, 8, E
fire digits: 0, 4, 8
earth digits: 1, 5, 9
air digits: 2, 6, X
water digits: 3, 7, E
English numerals of powers of 10:
1 one
10 dozen / onety
100 gross / hundred
1000 grossiad / thousand
Sets of 3 zeros
(n) (n-illion) (number of zeros: 3n+3) (value: 103n+3)
1 million 6 (1,000,000)
2 billion 9 (1,000,000,000)
3 trillion 10 (1,000,000,000,000)
4 quadrillion 13 (1,000,000,000,000,000)
5 quintillion 16 (1,000,000,000,000,000,000)
6 sextillion 19 (1,000,000,000,000,000,000,000)
7 septillion 20 (1,000,000,000,000,000,000,000,000)
8 octillion 23 (1,000,000,000,000,000,000,000,000,000)
9 nonillion 26 (1,000,000,000,000,000,000,000,000,000,000)
X dekrillion 29 (1,000,000,000,000,000,000,000,000,000,000,000)
E elpillion 30 (1,000,000,000,000,000,000,000,000,000,000,000,000)
10 dozillion 33 (1,000,000,000,000,000,000,000,000,000,000,000,000,000)
11 undozillion 36 (etc...)
12 duodozillion 39
13 tredozillion 40
14 quattuordozillion 43
15 quindozillion 46
16 sexdozillion 49
17 septendozillion 50
18 octodozillion 53
19 novemdozillion 56
1X dekradozillion 59
1E elpindozillion 60
20 vigintillion 63
21 unvigintillion 66
22 duovigintillion 69
23 trevigintillion 70
24 quattuorvigintillion 73
25 quinvigintillion 76
26 sexvigintillion 79
27 septenvigintillion 80
28 octovigintillion 83
29 novemvigintillion 86
2X dekravigintillion 89
2E elpinvigintillion 90
30 trigintillion 93
31 untrigintillion 96
32 duotrigintillion 99
33 tretrigintillion X0
34 quattuortrigintillion X3
35 quintrigintillion X6
36 sextrigintillion X9
37 septentrigintillion E0
38 octotrigintillion E3
39 novemtrigintillion E6
3X dekratrigintillion E9
3E elpintrigintillion 100
40 quadragintillion 103
41 unquadragintillion 106
42 duoquadragintillion 109
43 trequadragintillion 110
44 quattuorquadragintillion 113
45 quinquadragintillion 116
46 sexquadragintillion 119
47 septenquadragintillion 120
48 octoquadragintillion 123
49 novemquadragintillion 126
4X dekraquadragintillion 129
4E elpinquadragintillion 130
50 quinquagintillion 133
51 unquinquagintillion 136
52 duoquinquagintillion 139
53 trequinquagintillion 140
54 quattuorquinquagintillion 143
55 quinquinquagintillion 146
56 sexquinquagintillion 149
57 septenquinquagintillion 150
58 octoquinquagintillion 153
59 novemquinquagintillion 156
5X dekraquinquagintillion 159
5E elpinquinquagintillion 160
60 sexagintillion 163
61 unsexagintillion 166
62 duosexagintillion 169
63 tresexagintillion 170
64 quattuorsexagintillion 173
65 quinsexagintillion 176
66 sexsexagintillion 179
67 septensexagintillion 180
68 octosexagintillion 183
69 novemsexagintillion 186
6X dekrasexagintillion 189
6E elpinsexagintillion 190
70 septuagintillion 193
71 unseptuagintillion 196
72 duoseptuagintillion 199
73 treseptuagintillion 1X0
74 quattuorseptuagintillion 1X3
75 quinseptuagintillion 1X6
76 sexseptuagintillion 1X9
77 septenseptuagintillion 1E0
78 octoseptuagintillion 1E3
79 novemseptuagintillion 1E6
7X dekraseptuagintillion 1E9
7E elpinseptuagintillion 200
80 octogintillion 203
81 unoctogintillion 206
82 duooctogintillion 209
83 treoctogintillion 210
84 quattuoroctogintillion 213
85 quinoctogintillion 216
86 sexoctogintillion 219
87 septenoctogintillion 220
88 octooctogintillion 223
89 novemoctogintillion 226
8X dekraoctogintillion 229
8E elpinoctogintillion 230
90 nonagintillion 233
91 unnonagintillion 236
92 duononagintillion 239
93 trenonagintillion 240
94 quattuornonagintillion 243
95 quinnonagintillion 246
96 sexnonagintillion 249
97 septennonagintillion 250
98 octononagintillion 253
99 novemnonagintillion 256
9X dekranonagintillion 259
9E elpinnonagintillion 260
X0 dekragintillion 263
X1 undekragintillion 266
X2 duodekragintillion 269
X3 tredekragintillion 270
X4 quattuordekragintillion 273
X5 quindekragintillion 276
X6 sexdekragintillion 279
X7 septendekragintillion 280
X8 octodekragintillion 283
X9 novemdekragintillion 286
XX dekradekragintillion 289
XE elpindekragintillion 290
E0 elpagintillion 293
E1 unelpagintillion 296
E2 duoelpagintillion 299
E3 treelpagintillion 2X0
E4 quattuorelpagintillion 2X3
E5 quinelpagintillion 2X6
E6 sexelpagintillion 2X9
E7 septenelpagintillion 2E0
E8 octoelpagintillion 2E3
E9 novemelpagintillion 2E6
EX dekraelpagintillion 2E9
EE elpinelpagintillion 300
100 centillion 303
(there is no consistent and widely accepted way to extend cardinals beyond centillion)
(numbers not coprime to 10 (dozen) only have bent lines, number coprime to 10 (dozen) only have straight lines)
(this is just digit notation, for the sound, k- for digits divisible by 3, t- for digits == 1 mod 3, p- for digits == 2 mod 3, -o (using Japanese sound) for digits divisible by 4, -i for digits == 1 mod 4, -u for digits == 2 mod 4, -e for digits == 3 mod 4)
(Japanese sound) 0 = ko, 1 = ti (just “ti”, not “chi”), 2 = pu, 3 = ke, 4 = to, 5 = pi, 6 = ku, 7 = te, 8 = po, 9 = ki, X = tu (just “tu”, not “tsu”), E = pe
(digit d rotating half turn is digit 10−d (0 is still 0), digit d mirroring left-right is digit d±6 (choose +/− to let the result number between 0 and 10) (0 is still 0, 6 is still 6), digit d mirroring up-down is digit 6−d (when 0<d<6) or 16−d (when 6<d<10) (0 is still 0, 6 is still 6))
In this digit notation:
Strobogrammatic numbers: 0, 6, 1E, 2X, 39, 48, 57, 66, 75, 84, 93, X2, E1, 10E, 16E, 20X, 26X, 309, 369, 408, 468, 507, 567, 606, 666, 705, 765, 804, 864, 903, 963, X02, X62, E01, E61, 100E, 11EE, 12XE, 139E, 148E, 157E, 166E, 175E, 184E, 193E, 1X2E, 1E1E, 200X, 21EX, 22XX, 239X, 248X, 257X, 266X, 275X, 284X, 293X, 2X2X, 2E1X, 3009, 31E9, 32X9, 3399, 3489, 3579, 3669, 3759, 3849, 3939, 3X29, 3E19, 4008, 41E8, 42X8, 4398, 4488, 4578, 4668, 4758, 4848, 4938, 4X28, 4E18, 5007, 51E7, 52X7, 5397, 5487, 5577, 5667, 5757, 5847, 5937, 5X27, 5E17, 6006, 61E6, 62X6, 6396, 6486, 6576, 6666, 6756, 6846, 6936, 6X26, 6E16, 7005, 71E5, 72X5, 7395, 7485, 7575, 7665, 7755, 7845, 7935, 7X25, 7E15, 8004, 81E4, 82X4, 8394, 8484, 8574, 8664, 8754, 8844, 8934, 8X24, 8E14, 9003, 91E3, 92X3, 9393, 9483, 9573, 9663, 9753, 9843, 9933, 9X23, 9E13, X002, X1E2, X2X2, X392, X482, X572, X662, X752, X842, X932, XX22, XE12, E001, E1E1, E2X1, E391, E481, E571, E661, E751, E841, E931, EX21, EE11, ...
Strobogrammatic primes: 1E, 57, 75, 16E, 507, 705, E61, 148E, 157E, 184E, 1X2E, 5007, 51E7, 5397, 5487, 5X27, 5E17, 7005, 71E5, 7395, 7665, 7E15, E001, E2X1, E481, E571, E931, 1000E, 1060E, 1369E, 1468E, 1666E, 1765E, 1963E, 50007, 50607, 516E7, 526X7, 53697, 57657, 58647, 70605, 716E5, 7E615, E0001, E0601, E7651, 10570E, 10930E, 10X20E, 1139EE, 1184EE, 11X2EE, 1293XE, 12X2XE, 13489E, 13E19E, 14008E, 15007E, 152X7E, 15757E, 15847E, 16396E, 16576E, 17575E, 17665E, 17E15E, 18004E, 18664E, 18E14E, 19003E, 192X3E, 19483E, 1X482E, 1X842E, 1XE12E, 1E2X1E, 1EX21E, 500007, 501E07, 502X07, 503907, 50E107, 5148E7, 51E1E7, 5200X7, 52X2X7, 530097, 533997, 539397, 53X297, 541E87, 543987, 551E77, 557577, 55X277, 564867, 566667, 56X267, 56E167, 573957, 574857, 579357, 57X257, 580047, 582X47, 584847, 588447, 594837, 59E137, 5X1E27, 5X3927, 5E1E17, 5E6617, 701E05, 708405, 709305, 70X205, 70E105, 712XE5, 7284X5, 735795, 736695, 739395, 73X295, 744885, 74E185, 752X75, 753975, 762X65, 76E165, 775755, 782X45, 789345, 78X245, 790035, 791E35, 793935, 799335, 79E135, 7X3925, 7X5725, 7E6615, 7E8415, E05701, E09301, E0X201, E11EE1, E157E1, E184E1, E248X1, E257X1, E275X1, E30091, E33991, E35791, E40081, E44881, E50071, E51E71, E54871, E56671, E62X61, E65761, E67561, E71E51, E74851, E75751, E81E41, E98431, EX0021, EX4821, EE1E11, EE2X11, EE3911, EE8411, ...
Strobogrammatic squares: 0, 84, 309, 804, 87E154, ...
Horizontally symmetric numbers: 0, 3, 6, 9, 15, 24, 33, 42, 51, 66, 7E, 8X, 99, X8, E7, 105, 135, 165, 195, 204, 234, 264, 294, 303, 333, 363, 393, 402, 432, 462, 492, 501, 531, 561, 591, 606, 636, 666, 696, 70E, 73E, 76E, 79E, 80X, 83X, 86X, 89X, 909, 939, 969, 999, X08, X38, X68, X98, E07, E37, E67, E97, 1005, 1155, 1245, 1335, 1425, 1515, 1665, 17E5, 18X5, 1995, 1X85, 1E75, 2004, 2154, 2244, 2334, 2424, 2514, 2664, 27E4, 28X4, 2994, 2X84, 2E74, 3003, 3153, 3243, 3333, 3423, 3513, 3663, 37E3, 38X3, 3993, 3X83, 3E73, 4002, 4152, 4242, 4332, 4422, 4512, 4662, 47E2, 48X2, 4992, 4X82, 4E72, 5001, 5151, 5241, 5331, 5421, 5511, 5661, 57E1, 58X1, 5991, 5X81, 5E71, 6006, 6156, 6246, 6336, 6426, 6516, 6666, 67E6, 68X6, 6996, 6X86, 6E76, 700E, 715E, 724E, 733E, 742E, 751E, 766E, 77EE, 78XE, 799E, 7X8E, 7E7E, 800X, 815X, 824X, 833X, 842X, 851X, 866X, 87EX, 88XX, 899X, 8X8X, 8E7X, 9009, 9159, 9249, 9339, 9429, 9519, 9669, 97E9, 98X9, 9999, 9X89, 9E79, X008, X158, X248, X338, X428, X518, X668, X7E8, X8X8, X998, XX88, XE78, E007, E157, E247, E337, E427, E517, E667, E7E7, E8X7, E997, EX87, EE77, ...
Horizontally symmetric primes: 3, 15, 51, E7, 105, 195, 531, 591, 70E, 76E, E37, E67, E97, 1005, 1245, 1425, 1995, 5001, 5331, 5421, 57E1, 5X81, 700E, 766E, E157, E337, E8X7, EX87, 10905, 11355, 12345, 12945, 13335, 14325, 14625, 15915, 16065, 170E5, 183X5, 19395, 1E675, 50001, 50301, 54321, 54921, 56361, 573E1, 583X1, 59391, 5X681, 5E671, 7204E, 7234E, 7264E, 7303E, 7363E, 7432E, 7531E, 7666E, 7696E, 773EE, 783XE, 789XE, 7999E, 7X38E, 7X68E, 7E97E, E0007, E0907, E2047, E3037, E3637, E5617, E73E7, E83X7, E86X7, E89X7, EX387, EX687, 102405, 108X05, 114255, 118X55, 123345, 128X45, 133335, 134235, 146625, 148X25, 14X825, 152415, 155115, 156615, 161565, 163365, 16X865, 1742E5, 177EE5, 1815X5, 1866X5, 1899X5, 190095, 191595, 192495, 194295, 197E95, 1X0085, 1X5185, 1X9985, 1E0075, 1E3375, 1E6675, 1E7E75, 1E8X75, 1EX875, 502401, 506601, 50E701, 513351, 514251, 517E51, 518X51, 51X851, 522441, 52X841, 538X31, 539931, 53X831, 544221, 546621, 557E11, 55E711, 568X61, 5715E1, 578XE1, 57X8E1, 5800X1, 5815X1, 5842X1, 5851X1, 5866X1, 5899X1, 58X8X1, 594291, 5X4281, 5XE781, 5EX871, 5EE771, 70X80E, 71155E, 71245E, 717E5E, 71X85E, 71E75E, 72154E, 72514E, 72664E, 728X4E, 72E74E, 73003E, 73153E, 738X3E, 73993E, 74332E, 74E72E, 75421E, 75661E, 75E71E, 76426E, 768X6E, 7751EE, 788XXE, 79009E, 79249E, 79429E, 79X89E, 7X158E, 7X248E, 7X338E, 7XE78E, 7E517E, 7E667E, 7E8X7E, 7EE77E, E04207, E06607, E08X07, E11557, E12457, E18X57, E24247, E28X47, E34237, E35137, E39937, E40027, E44227, E46627, E54217, E62467, E68X67, E733E7, E77EE7, E78XE7, E88XX7, E8X8X7, E9X897, EX5187, EX6687, EX9987, EXX887, EE1577, EE5177, ...
Dozenal Sudoku (Sudoku with 10×10 square divided into 10 4×3 rectangles, and numbers 0 to E (each has 10 times))
Dozenal 1010! (1010! with 10×10 square and fixed polyomino with 1 to 6 cells (monomino through hexomino) plus the 4×2 rectangle (an octomino), the 3×3 square (a nonomino), and the 4×3 rectangle (a dozomino), one time the player get 3 polyominoes)
Dozenal Kakuro (the allowed numbers are 1 to E, and no repeating digit in the same column/row)
Dozenal KenKen (KenKen with 10×10 square. the allowed numbers are 0 to E)
Dozenal Nonogram (Nonogram with 10×10 square)
Dozenal Futoshiki (Futoshiki with 10×10 square and numbers 0 to E (each has 10 times))
Dozenal Hidato (Hidato with 10×10 square and numbers 0 to EE)
Dozenal 10^10 (the original game is 2^E, only two same numbers in a column/row can be added, and start with the numbers 2 and 4, and a player who get 2^E can win, but in the new 10^10 game, it is a 10×10 square, and 2,3,4,6,10 same numbers in a column/row can be added (if the result number does not divide 10^10, then these numbers cannot be added), and start with the numbers 1,2,3,4,6,10, and a player who get 10^10 can win)